Double 'bulge' tide explanation
If you look at any explanation of tides you will see a diagram that looks something like fig.1 which shows the tides represented as two bulges of water – one directly under the Moon and another on the opposite side of the Earth. Most people appreciate that tides are caused by gravitational forces and so can understand the moon-side bulge; however the second bulge is often a cause of confusion. This article attempts to explain why there are two bulges.

Before we look at this in detail, we need to clarify a few points.
1. The Moon does not orbit around the Earth. “What!” I hear you cry, “of course it does – they taught me that at school.” In reality, the moon and earth both orbit around each other, or more precisely around their common centre of mass which happens to lie inside the Earth.
2. There are two forces acting on the Earth’s oceans and seas: gravity due to the presence of the
moon and a centrifugal force due to the rotational motion of the Earth-Moon system. At this point some scientists will argue that centrifugal forces are fictitious. If you are looking at the system from a fixed point in space (an inertial or non-accelerating frame of reference) then this is correct. However, if you are in a rotating frame of reference, the force is indeed real. Try driving
fast around a tight bend and tell me you can’t feel a force pushing you to the side. You are in the rotating frame of reference hence the force can be felt.
3. In the discussion about what causes the two bulges of water you must completely ignore the rotation of the Earth on its axis (the 24 hr daily rotation). Any talk of rotation refers to the 27.3 day rotation of the Earth and Moon about their common centre of mass.
So let’s look at the forces acting at different points on the Earth’s surface. In fig 2, the blue arrows are the forces due to the gravitational attraction of the moon. The red arrows are the centrifugal
force experienced by bodies on the earth. The size of the arrow approximates the magnitude of the force. The scale is highly exaggerated.
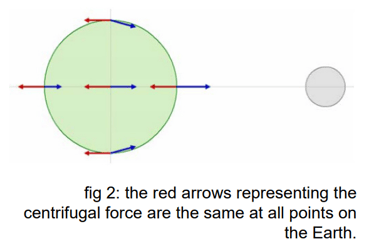
Firstly you will notice that all the red arrows are exactly the same length. This is because the 27.3 day rotation of the earth about the Earth-Moon centre of mass causes every point on the earth to move in a circle of equal radius at equal speed. Therefore the centrifugal force is the same for all points on the Earth.
Try this analogy: take something round like a roll of sticky tape, put it on the desk and move it
in small circles (not rotating it, just moving the whole thing it in a circular manner). You will see that every point on the object moves in a circle of equal radius and the same speed.
Now let’s look at the gravitation pull experienced by objects on the Earth due to the Moon. The
magnitude and direction of this force will be different at different points on the Earth’s surface. The equation for the magnitude of gravitational force between two objects is:
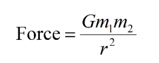
m1 and m2 is the mass of the two objects, and r is the distance between them
This shows that the strength of the force is inversely proportional to the square of the distance between them (i.e. if the distance doubles, the force is reduced by a factor of four). Therefore the magnitude will be slightly greater on the side of the Earth nearest the Moon than on the side of the Earth facing away from the moon as it is further away.
The direction of the force is always towards the centre of the Moon. The resultant force that acts on the water in our oceans (which we shall call the tidal force) is a combination of these two forces. If you add them together you get the small green arrows shown in fig 3 (again,
not to scale).
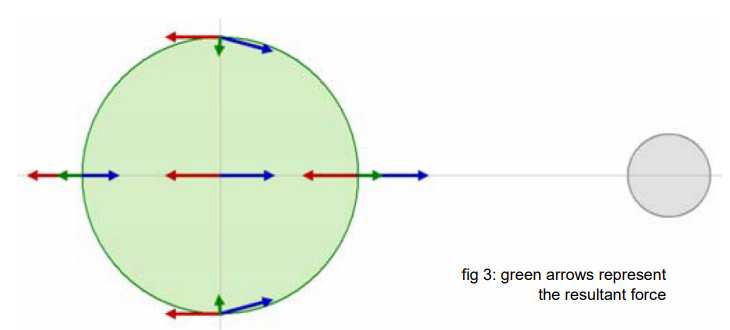
The force of gravity at the centre of the Earth balances the centrifugal force (i.e. is equal in magnitude but in exactly the opposite direction, therefore no net force)
In fact the diagram below shows the tidal forces and numerous points on the Earth’s surface.
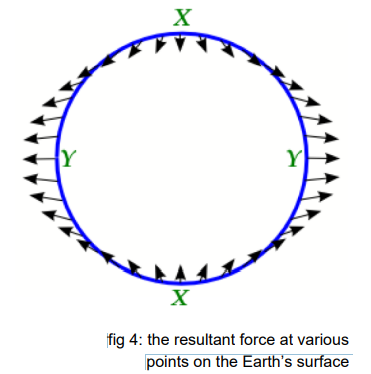
If a force is acting directly towards the centre of the Earth (as at the points marked X) or directly away from the Earth (points marked Y), then there is no force acting horizontally at the Earth’s surface (it is all either into or away from the Earth). Therefore there is no force to cause any movement of water across the Earth’s surface.
However at points in between, there is going to be some component of the force which does
act horizontally and this will cause a movement of water – an obvious requirement for tides.
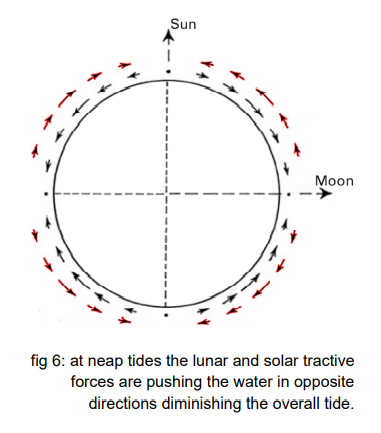
These distribution of these horizontal components of the tidal force (known as the tractive force) is shown below.
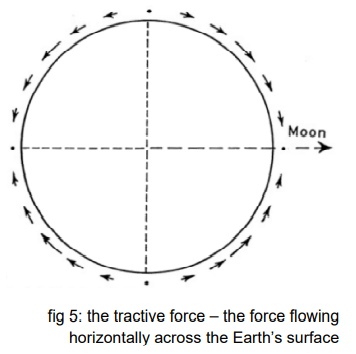
You can probably see now why the bulges occur both on the side of the Earth facing the Moon and on the side of the Earth away from the Moon.
The explanation above takes into account just the Moon, although the sun also creates its own double bulge. When the Earth, Moon and Sun are in line (at full and new Moon), the position of the lunar and solar bulges coincides and the tides are higher than average. These are called spring tides.
When the Earth, Moon and Sun form a right-angle (first and third quarter), the effect is for them to partially cancel each other out and create what are known as neap tides.